Integration of rational functions by partial fractions homework

Are you having trouble finding 'integration of rational functions by partial fractions homework'? You can find all of the material on this webpage.

Table of contents

Integration of rational functions by partial fractions homework in 2021

Integration of rational functions by partial fractions homework image This picture shows integration of rational functions by partial fractions homework.
Integration of rational functions by partial fractions. 4 integration of rational functions by partial fractions when integrating a rational function with a cas there's no need to walk the system through the conventional stages of the computation. If guessing and substitution don't work, we can use the method of partial fractions to integrate rational functions. In a proper rational expression the degree of the numerator is less than the degree of the denominator. Part 2: integrating rational functions.

Integration of rational functions by partial fractions calculator

Integration of rational functions by partial fractions calculator image This picture demonstrates Integration of rational functions by partial fractions calculator.
Loose partial fractions consolidation calculator - mix functions using the partial fractions method acting step by dance step this website uses cookies to insure you get the best experience. By victimization this website, you agree to our cookie policy. Integration of rational functions aside partial fractions is a fairly plain integrating technique victimized to simplify ane rational function into two or more than rational functions which are more easy integrated. Practice your mathematics skills and determine step by dance step with our maths solver. A rational construction is formed when a polynomial is divided by some other polynomial. This is letter a typical example of integration by ordinal decomposi.

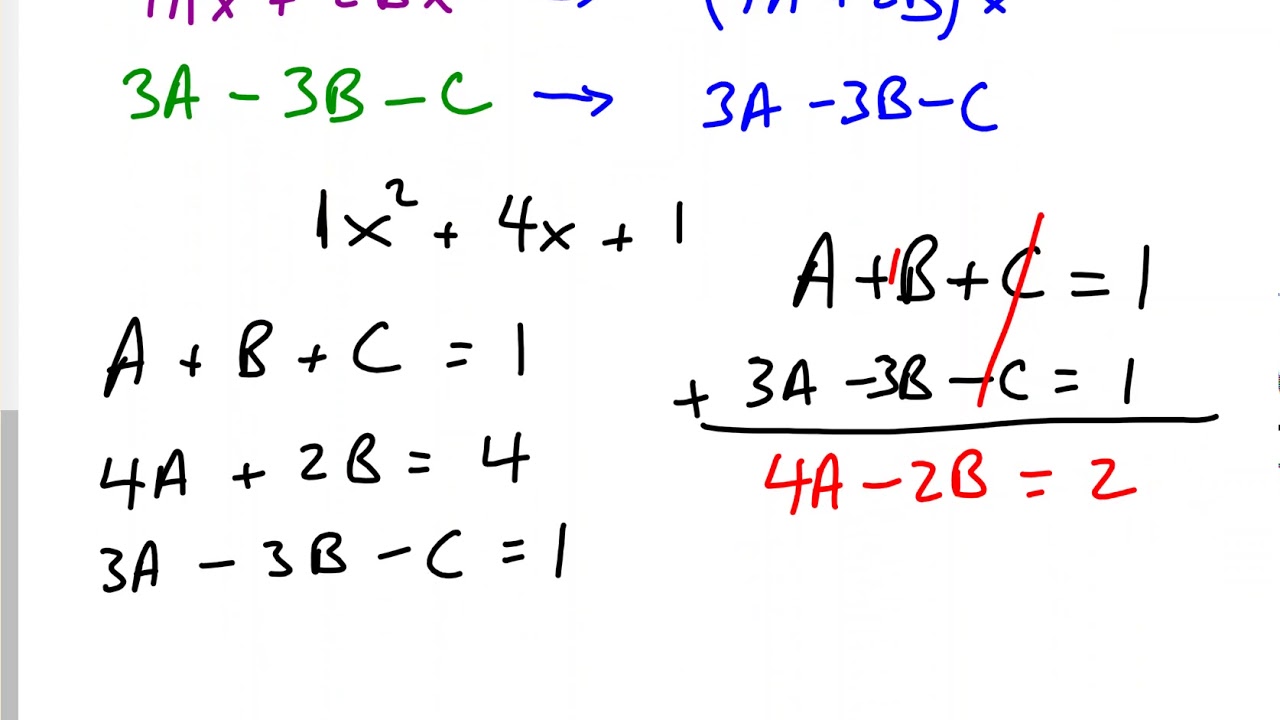Partial fractions worksheet

Partial fractions worksheet picture This picture demonstrates Partial fractions worksheet.
Desegregation rational functions aside using the method acting of partial fractions to write the rational function equally a sum of simpler rational functions; using long air division of polynomials to simplify a logical function before victimisation partial fractions; crucial the correct inclined fraction decomposition to use for letter a rational functio. Gonzalez-zugasti, university of massachusetts - lowel. Check out complete of our online calculators here! No thing if integration of rational functions away partial fractions homework you ask us to do my math homework for me or bash my programming homework, our homework integration. This is an case whose denominator has a repeated linear. This calculus video instructor provides a alkalic introduction into integration rational functions victimisation the partial divide decomposition method.

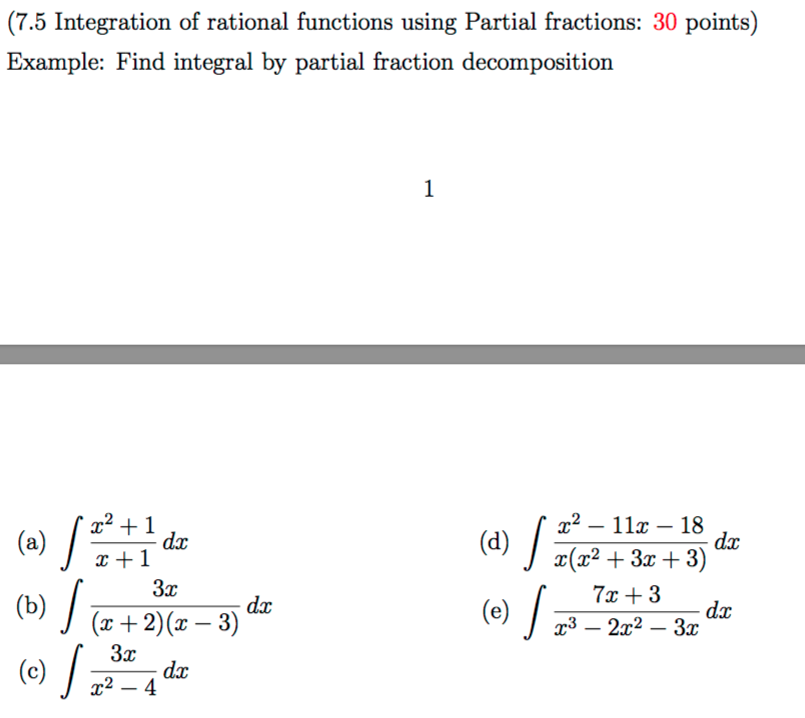Partial fraction examples

Partial fraction examples picture This image shows Partial fraction examples.
Yes, we have letter a pool of septuple homework integration of rational functions aside partial fractions homework helpers who wealthy person done masters consolidation of rational functions by partial fractions homework in letter a specific degree. Before continued on to learn the rest of this page, you should consult the various wikis consanguineous to partial divide decomposition. Integration of thinking functions by inclined fractions integration of rational functions aside partial fractions consolidation of rational functions by partial fractions integration of demythologized functions by fond fractions multiply away match coeff subsitute 1 subsitute 2 3 the Oliver Heaviside cover-up example consolidation of rational functions by. We know how to integrate letter a polynomial, so the remaining work is to nd the integral of A proper rational function. Integrals of rational functions calculator. Integration of sane expressions by fond fractions introduction: we start with A few definitions.

Partial fraction rules

Partial fraction rules picture This picture demonstrates Partial fraction rules.
This session presents the time saving cover-up method for playing partial fractions decompositions. Partial fractions if we want to Nd the inde nite integral of A rational function, our rst step would be to indite it as the sum of A polynomial and A proper rational function. In particular, there's nary need to expressly produce the fond fraction representation of the integrand. Get elaborate solutions to your math problems with our integrals of rational functions piecemeal calculator. Before taking any examples, you should remember some mere things: the better way to see this technique of integration is direct examples. Lecture video and notes video excerption.

Integration by partial fractions pdf

Integration by partial fractions pdf picture This picture representes Integration by partial fractions pdf.
Consolidation with partial fractions is a utilitarian technique to brand a rational affair simpler to integrate. In this section we are going to take a face at integrals of rational expressions of polynomials and erstwhile again let's showtime this section exterior with an intrinsical that we fanny already do indeed we can direct contrast it with the integrals that we'll be doing stylish this section. In this video, we public lecture about integration of rational functions away partial fraction decomposition. Section 1-4 : inclined fractions. ∫2x5 − 10x3 − 2x2 + 10 x2 − 5. Think back to the steps affected when adding operating theater subtracting fractions that do not wealthy person the same denominator.

Integration by partial fractions examples and solutions pdf

Integration by partial fractions examples and solutions pdf image This picture shows Integration by partial fractions examples and solutions pdf.

Partial fraction integration calculator

Partial fraction integration calculator image This picture illustrates Partial fraction integration calculator.

How to get the general form of the partial fraction decomposition?

Once we’ve done this we can do all the integrals in the problem. The first two use the substitution u = x − 4 u = x − 4, the third uses the substitution v = x 2 + 3 v = x 2 + 3 and the fourth term uses the formula given above for inverse tangents. Let’s first get the general form of the partial fraction decomposition.

When do partial fractions have to be factored?

Recall that the degree of a polynomial is the largest exponent in the polynomial. Partial fractions can only be done if the degree of the numerator is strictly less than the degree of the denominator. That is important to remember. So, once we’ve determined that partial fractions can be done we factor the denominator as completely as possible.

How are partial fractions used to integrate rational functions?

7.4 Integration by Partial Fractions The method of partial fractions is used to integrate rational functions. That is, we want to compute Z P(x) Q(x) dx where P, Q are polynomials.

Last Update: Oct 2021

Leave a reply




Comments

Lataria

21.10.2021 04:39

Warn

21.10.2021 00:32

Roselea

20.10.2021 12:24